Trochaic Substitutions in Iambic Verse

I fear I am about to give you a lecture. Blame Alon, he asked for it (In the comments of this post. Sort of).

First things first. When we speak English, some syllables have stronger emphasis placed on them than others. By this I do not mean the same sort of emphasis that we would denote by italics. I mean the natural variations in stress that create the rhythm of our speech. Following Stephen Fry's convention in this lovely book, when I want to show you which syllables are stressed I shall write the stressed syllables in bold, like this:

The natural variations in stress that create the rhythm of our speech.

An iamb is a weak (unstressed) syllable followed by a strong (stressed) syllable. In ordinary speech I might be likely to pronounce it iamb -- but don't. As a way of remembering it, stress the second syllable: iamb. Iamb, iamb, iamb, iamb. That's the rhythm of iambic verse.

Fair youth, beneath the trees, thou canst not leave
Thy song, nor ever can those trees be bare;
Bold Lover, never, never canst thou kiss,
Though winning near the goal -- yet do not grieve;
She cannot fade, though thou hast not thy bliss,
For ever wilt thou love, and she be fair!

(Keats, Ode on a Grecian Urn)

It is worth noting that if you merely read a poem in your head, you are less likely to take note of which syllables are strong and which ones are weak. This is one reason (and not the only one) for getting into the habit of always reading poems aloud. Even just whispering the poem makes a real difference to what you can get out of it. So, please, when I quote lines of poetry in this post, read them aloud to yourself. And if you will do me the courtesy of reading my humble poems aloud when I post them, I shall bless you as a considerate and thoughtful reader.

The excerpt from Ode on a Grecian Urn that I gave above is written in iambic pentameter. That is to say, there are five iambic 'feet' in each line; five sets of 'weak-strong'. Iambic tetrameter, with four iambs per line, is also quite common:

They fuck you up, your mum and dad.
They may not mean to, but they do.
They fill you with the faults they had
And make some extra, just for you.

(Philip Larkin, This Be The Verse)

A trochee is sort of the opposite of an iamb: 'strong-weak' rather than 'weak-strong'. Trochaic verse is less common than iambic verse, but there are certainly examples out there. Longfellow's Hiawatha, for example, is written in trochaic tetrameter (the name of the title character may have had something to do with that).

And the smoke rose slowly, slowly,
Through the tranquil air of morning,
First a single line of darkness,
Then a denser, bluer vapor,
Then a snow-white cloud unfolding,
Like the tree-tops of the forest,
Ever rising, rising, rising,
Till it touched the top of heaven . . . (link)

Longfellow wrote Hiawatha in huge sentences; they kind of drag on, sometimes, in my opinion. However, if I was writing trochaic verse I think I would have a hard time not doing that, myself. Trochees are not very, well, conclusive; they end weakly. Iambic verse, by contrast, ends on a strong syllable and, as a result, tends to sound stronger as a whole.

Within iambic verse, a trochaic substitution occurs when you substitute a trochee for one of your iambic feet. It can occur at the beginning of a line, like this:

Here with a Loaf of Bread beneath the Bough . . .

(Edward Fitzgerald, The Rubaiyat of Omar Khayyam)

It can also occur in the middle of a line, at the beginning of a new phrase, like this:

To be or not to be: that is the question.

(Don't get confused by the extra syllable at the end, there; it's called a feminine ending, and it's another perfectly common variation)

A trochaic substitution in the middle of a line forces us to have two stressed syllables next to each other. This naturally creates a tendency to pause between the two strong syllables. As a result, it's generally bad form to use a trochaic substitution in the middle of a line in a situation where the pause would not be natural. This rule about where trochaic substitutions are allowed to go is nearly universal. However, the effects that can be produced by trochaic substitutions within those limits are startlingly varied.

A trochaic substitution can be a way to start things with a bang:

Out flew the web and floated wide;
The mirror crack'd from side to side;
"The curse is come upon me," cried
The Lady of Shalott.

(Tennyson, The Lady of Shalott)

Trochaic substitutions can fit nicely into a strong, purposeful rhythm, as in the conclusion of this poem by Wilfred Owen where he explains why he isn't writing jolly little poems about the ways in which war isn't so bad after all:

You shall not hear their mirth:
You shall not come to think them well content
By any jest of mine. These men are worth
Your tears. You are not worth their merriment.

(Wilfred Owen, Apologia Pro Poemate Meo)

Trochaic substitutions can be used in dramatic verse to break the rhythm of a character's speech to show agitation. There are several good examples in Macbeth, Act I, Scene VII. Here's Lady Macbeth, berating her husband with trochaic substitutions all over the place:

Was the hope drunk
Wherein you dress'd yourself? hath it slept since?
And wakes it now, to look so green and pale
At what it did so freely? From this time
Such I account thy love.

My favourite trochaic substitution, though, is nearly invisible. It's a lilt, a beauty mark, at the beginning of the fourth line of the following poem. Since the metre flows naturally on its own, I won't spoil the elegance of it by crudely marking it out.

She walks in beauty, like the night
Of cloudless climes and starry skies;
And all that's best of dark and bright
Meets in her aspect and her eyes:

(Byron, She Walks In Beauty)

Would you rather be a good enough poet to write that, or would you rather be pretty enough to have that written about you? I can't decide. Not that I would be foolish enough to have anything to do with mister 'mad, bad and dangerous to know' if he was still alive, but he did write awfully sexy poetry.

If Emily Dickinson is writing it, a trochaic substitution can even be soft and lingering:

Hope is the thing with feathers
that perches in the soul . . . (link)

It's startling, but entirely typical of poetry, that the rules can be so strict, and the results so varied. I find that knowing something of the ways in which metre can vary makes a big difference to the way I read poetry. In Shakespeare, the metre can sometimes amount to a stage direction to say a line in a particular way. In Wilfred Owen's most famous poem, Dulce et Decorum Est, I read it again after I'd learned to read metre and couldn't believe I had never noticed before the way the metre sputters and dies in the final line. When Ebonmuse posted some excerpts from Wallace Stevens' Sunday Morning, I knew full well I wouldn't have read them in the same even, powerful way if I wasn't sorting each pentametric line into iambic beats (with the occasional trochaic one).

It's worth knowing.

'Streetcar' and Censorship.

Yesterday, I finally cracked. I was on my way home from work and I passed the DVD rental place, wandered in and, well, somehow A Streetcar Named Desire was just what I felt like seeing. I’d been holding off for a while. I had my reasons.

I saw a stage production of The Glass Menagerie a few months ago. It was incredible. We don’t study Tennessee Williams in high school over here. Shakespeare, sure, every year at least once, and if you don’t hit Steinbeck there’s a good chance someone’ll hand you To Kill A Mockingbird, but Tennessee Williams isn’t on anybody’s radar for exam fodder. So I think it was somewhere around the point in Tom’s opening speech where he refers to the fiddler in the wings that I became aware of -- became awed by -- the autheticity of the voice. It was intimate. It was elegantly constructed. It had a rough edge. It was incontrovertibly American. This was poetry created from the surrounding voice. It couldn’t rely on literary conventions to mark it out as art. It was the real deal.

I was so glad I had never had to write English assignments on it.

Of course I wanted more. Still, I was wary of watching Tennessee Williams on film. Having seen The Glass Menagerie in the theatre, I honestly couldn’t imagine how you could possibly make a film out of it. The way it uses the stage setting is too beautiful, the way it doesn’t entirely pretend to be real. It’s nostalgic -- it’s a stage, an illusion -- it’s intimate, but it doesn’t pretend to be entirely real. How could you possibly capture that on film? Why would you want to?

I didn’t know if his later plays had anything of the same effect. But I couldn’t help worrying that film really couldn’t capture Tennessee Williams, and that I might be spoiling a future opportunity to see the real thing on stage. When it came to Streetcar, there was the plus side that Tennessee Williams had had a hand in the screenplay. If he thought it was okay, maybe it would be! On the other hand, though, there was the censorship issue. Did I really want to see a version that might have had its teeth pulled by prudish fifties industry watchdogs?

(Spoilers follow because I can’t shut up about important plot details.)

Well, I’ve seen it, no going back now, and I think it was probably worth it. The censorship does pose problems, but the actors are brilliant. It’s the film that made Marlon Brando’s name, of course, and Vivien Leigh is as good as you’d expect, but I was actually particularly impressed by Kim Hunter as Stella. She deserved that Oscar. She was a great contrast to Leigh’s pale waif-like Blanche, and I loved the complexity of emotion in her sulky mouth. I was watching the director’s cut, which meant we had the nice close-up in that scene where she goes back down to Stanley after he has hit her -- really, it’s a crying shame the League of Decency forced them to change that scene at the last minute. It was one of the most impressive sequences in the whole film, central to portraying Stella and Stanley’s relationship.

I’m glad I knew already that Blanche’s husband was homosexual. You could see perfectly well how the stuff she said about him applied to that situation (“He came to me for help, but I didn’t know that”). I’d have hated to be confused into thinking he was just oversensitive enough to kill himself over nothing.

It’s the end that I regret. When I saw Stella say to Stanley “Don’t you touch me,” I thought Yeah, right, how long is that going to last? Then she goes to her baby and says “We’re not going back in there, not ever,” and I thought Oh. Really? That’s nice. Gosh. So it’s a proper tragic ending, huh? Everyone loses, but at least that includes the bad guy. Not so terrible, really!

They suckered me. I am ashamed of myself.

Those stuffy, long-dead bastards! See what they’ve done? I’ll never get that moment back. For, of course, in the original play, Stella doesn’t leave Stanley. She never would. She’s dependent on him emotionally and financially. There were no battered women’s shelters in those days, but even if there were, believing Blanche’s story would have required the courage to see her world fall apart. She wasn’t going to do that. And I knew it, I could see it, right up to the point where she did differently, and I was desperately afraid of my own reaction -- afraid that I’d think that Stella and Stanley’s love, such as it was, was the only good thing still standing, despite its rottenness -- afraid that I’d have to see in it a bitter hope that I couldn’t reject because it was the only thing left.

I wasn’t so much glad that Stella had left Stanley as I was glad that I wasn’t sorry for it. And I won’t get that moment back. I won’t know how I would have dealt with it if the stupid Hollywood Production Code hadn’t insisted that rape couldn’t go unpunished. Seriously, what did they think Tennessee Williams was writing? A play about the way we’d like the world to be? Did they think he was endorsing a world where sensitive homosexual boys could be driven to suicide, and where Blanche’s sexual behaviour could deny her the only possibility of happiness she had?

Perhaps they did endorse that. Stupid bastards.

Fry & Laurie on Patriotism

Since our English friend Tobe (sadly only intermittently posting these days) last posted a piece called "On Patriotism", I couldn't help thinking of him when I saw this sketch:



And yes, Stephen Fry and Hugh Laurie are atheists. But of course they are.

(I know, I know, danger zone: not posting for ages and then coming up with random YouTube videos. I've got ideas -- on this eclectic blog, who wouldn't -- but I keep second-guessing myself. I have been a little busy this week. I'll blame that.)

Monday

It's 6am. Rather be early this morning.
I think I'm in the wrong time zone. Last week I
well it's probably fine but I can't be sure she didn't
take it the wrong way completely. And yes I
forgot to shower yesterday and the day before I
spent the day in bed. Why didn't I
get out more? Bloody stupid! Spent too much
time on the internet. Should have called a friend.
Tense, relax. Don't try to smile.
Nothing to do but rise, and have a shower,
grab some food and take a bite
and step out hopefully. Let the world forgive
this wilted, weary woman; let me
return to reality, just a little bruised.

One of my favourite questions

(On the off chance that you don't understand a word of this but would quite like to, I've got half an explanation in the post below).

I always rather liked tutoring. It's definitely one of the better privileges of being a good maths student: small job, decent pay, not bad on a CV, and, best of all, regular opportunities to talk about mathematics. The best part of tutoring is definitely the questions people ask. One year, I had the lecturer's son in my tutorial group and he'd have a different question every week, guaranteed to be perceptive and usually quite hard to answer. That was fun. But you don't have to be a brilliant student to ask the question I'm about to explain here. You just have to be halfway interested and struggling with one of the trickier aspects of multivariate calculus.

The chain rule in the univariate case is fairly simple:



Because of the notation, it looks like a simple fraction cancellation, is easy to remember, and quickly becomes second nature. Moreover, you don't have too many variables to keep track of. You have y=f(x), x=g(t), and if you want to get really complicated you could note that y as a function of t would be written y=f(g(t)), and define f(g(t)) as being equal to some other, single function h(t), and that would be about it.

In the multivariate case, you have z=f(x,y), and then sooner or later you're going to be dealing with one of those 'change of variables' problems where you look at what z would be in terms of some other variables u and v instead. You have a problem like

and you'll be asked to figure out by first calculating , , and and then using the multivariate chain rule. So you look up the multivariate chain rule (or maybe you've got used to it by this stage) and it tells you nice and obviously that

and now you've got to work out and , which is a bummer, because you have u and v written as expressions in x and y, but what you need, in order to work out those partial derivatives, is the opposite. You're going to have to solve a simultaneous equation problem to get expressions that tell you x and y in terms of u and v.

Not everybody likes simultaneous equations, you know, especially when you have pesky 'u's and 'v's floating around instead of comfortable obvious numbers. At some point -- it always happens -- somebody is going to try to short cut.

"Look," they'll say...
"
"so
"
"and now I'll differentiate to get
".
"We're taking a partial derivative with respect to u so we hold everything else constant so we just treat x like a constant, right?"

Wrong.

The simplest way to point this out is to say "Look, x depends on u. It's equal to some function of u and v. So it's not constant as u changes and you can't take the partial derivative with respect to u and expect it to behave like a constant."

The conversation usually continues like this:

"So what do I have to do then?"
"Solve a simultaneous equation for x and y in terms of u and v. Get an expression for y that is only in terms of u and v, with no x terms, and then work out the derivative."
"Sigh."

Sometimes that's the end of it. Sometimes the student will probe further. It happens when you're talking to a student who bothers to think about things. Maybe it's the guy who discusses the problems he's working on with his friends and tries to work the tricky stuff out together. Or it'll be the young woman who always does her homework (all of it), and makes you feel embarrassed because you were never that dutiful, who abandons her usual meekness and asks, with a note of frustration in her voice,

"But why? Why can't I just do it the other way? I mean, I've got u, right, and I differentiate with respect to y holding the other stuff constant. Why does it matter whether the other stuff in the equation is x or v or whatever? I'm still basically taking the derivative of u in the y direction, right?"

I really like this question. I never asked it when I took that course and then I got really confused by basically the same issue when I started learning how to solve partial differential equations.

Remember, back in our univariate case, we had a function f that took you from x to y, a function g that took you from t to x, and a function h (equal to taking g, then f) that would take you straight from t to y. Now, it doesn't make sense to just talk about the derivative of y. There's a derivative of y with respect to x, and a derivative of y with respect to t. More to the point, though, the derivative basically depends on whether we are getting to y via the function f or via the function h.

It's the function we're using that counts. The derivative of y isn't a number associated with some numerical value of y, it's a number associated with how we pass through some point (x,y). We get that information about how we're passing through a particular point from the overall form of the function around that point. We call it "the derivative of y", but actually we're taking the derivative of a function that happens to be equal to y.

So, back up there, if you're taking "the derivative of y with respect to u", it matters whether the function that takes you from u to y includes x terms or if it's all in terms of u and v. It matters because you're changing the form of the function, and it's really the function that you take the derivative of.

You can also look at it this way. We say we're taking the derivative "in the u direction". In actual fact, though, the "u direction" really depends more on v that it does on u. The u direction is "the direction of increasing u", right? But there are lots of directions that increase u.



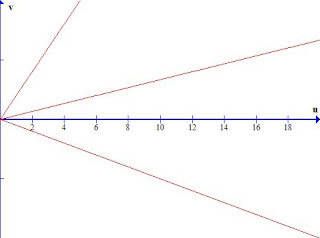
We could travel along any of those red lines and u would be increasing. But "the u direction" is the direction along which u increases without affecting v. And if the other variable that we're holding constant isn't v, if it's some other weird variable x that depends on a combination of u and v, then "the u direction" isn't "the direction of constant v" any more. It's "the direction of constant x". And that's different, so we get a different answer.

Make sense?

Lightning-Run Calculus: The Derivative

Bad news, oh ye who tremble in the presence of mathematical symbols! I had this cool idea for a maths post. And then I realised it involved multivariate calculus. And I didn't want to leave anybody out, so I thought I'd make a lightning-run post so that those who don't know any calculus and want to sort of have an idea of what I'm talking about should at least be able to get some of the ideas into your head.

I know that nobody who knows nothing of calculus is going to be able to pick up all the ideas presented in this post, all at once. I also know I'm not quite telling you everything you need to know to understand my post above. However, I figure that if you thought you vaguely understood something in the post I'm about to make after this one, you might be able to check in this post to see if you were thinking along the right lines.

At the bottom line, I just like having this stuff here for completeness.

I'm not quite sure if I can help anyone who is too afraid of algebra to understand the following:

Let m be the number of biscuits Mary ate. Let n be the number of biscuits Nathan ate. Between then, Mary and Nathan ate 12 biscuits in total. Therefore, m + n = 12.

You don't have to be able to figure out whan m and n are. In fact, in case you haven't noticed, you can't, because I haven't given you enough information. But I'm going to be working on the level of "getting the basic idea" rather than actually being able to calculate things, and I'm going to assume that you get the basic idea of how letters substitute for numbers in algebra. I'm also going to assume that you sort of get how we make a graph of a function like, say, . I mean, you might not be able to draw it off the top of your head, but I'm hoping you'd have a rough idea of what sort of a picture we're talking about, and you'd know that points on the curvy line drawn by the graph correspond to values of y, as calculated by that function, for given values of x.

Ready?

Derivatives

The derivative of a function at a point is the slope of the tangent line at that point. Here's a nice picture:


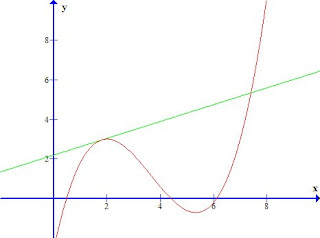
Picture created with the help of a piece of open-source software available here.

The red line is the function; the green line is the tangent line for the point x=1.8 . It just touches the graph of the function at that point without actually crossing it. As a result, at that point x=1.8, the function and the tangent line seem to be travelling in the same direction momentarily. By contrast, further to the right at x = roughly 7.2ish, the lines touch and cross each other. The green line is not a tangent to the red line at that point.

For the sake of accuracy, I'm forced to inform you that the tangent line at a particular point can cross the function at that very point. However, when that happens, it looks nothing like the right hand touching point above where the two lines cross at an angle. It looks like this:



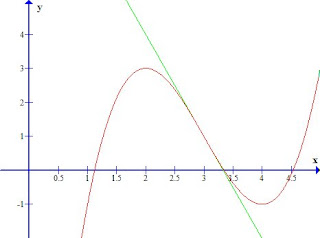
The basic idea is that the tangent line shows you what the function would do if it stopped bending at that point and just carried on in a straight line in the direction it was pointing at the time. It tells you what direction the function is going at that particular point. There's a perfectly specific and accurate way of defining it, but I'm not going to go there. That's not because it's all that terribly hard; I just don't want to overload you with too many concepts. If you want to understand more fully, find a calculus textbook and/or ask me enough questions in the comments section to convince me you're enthusiastic.

The slope of the tangent line is a number that measures how steep it is. A vertical line would have infinite slope; a horizontal line would have zero slope. If the slope of the tangent line is a big number (i.e. if the derivative is large) then both the function and its tangent line are travelling upwards very fast at that point. If the slope of the tangent line (= the derivative) is close to zero, then the function is nearly horizontal at that point. If it's a negative number, the function is travelling downwards. Got it? Don't worry, I won't be testing you later. You should still go through and check that you understand the idea here, though.

We write the derivative like this:

By which we mean:
"The derivative of y with respect to x"
or,
"What we get when we differentiate y with respect to x"
(Differentiation is the process that takes a function and gives you its derivative)
or, equivalently,
"How fast y changes as we increase x"

There is an explanation for this seemingly strange notation, but it would require me to go into the subject in greater depth than I intended for this post.

Why do we care about this? Well, any time we want to talk mathematically about how something is changing, derivatives are relevant. For example, if the exchange rate is rising fast, then the tangent line to the exchange rate will have a big slope. We can use the derivative of the exchange rate as a way of factoring changes in the exchange rate into an economic model. If we want to talk about how fast the position of an object is changing -- that is, if we want to talk about an object's speed -- then we need to look at the derivative of a graph of the object's position. If we want to talk about how fast the speed is changing -- that is, if we want to talk about acceleration -- we look at the derivative of the speed. That is, the derivative of the derivative of the position.

It's no coincidence that physics took off after Newton discovered calculus. There was a direct causal relationship (more than one causal relationship in more than one direction, perhaps). This is very useful maths. It's about as useful as mathematics could possibly get.

Partial derivatives

Sometimes, in multivariate calculus, you have a function that depends on more than one variable. For example, you might want a function that can tell you how much petrol a car will use based on how fast it is going and how much weight it is carrying. Or you might want to make a graph of altitude based on latitude and longitude. If you want some three-dimensional pictures of functions (graphed along the vertical z axis) that depend on two variables (graphed on the x and y axes), you can go here and type in some expressions in x and y into the box on the upper right that has 'z = ' to the left of it. Try looking at, you know, z = x^2+y^2+3*x^3 or something (That's computer keyboard speak for , in case you didn't know -- you use the * instead of an x for multiplication. I know I'm being really pedantic here but I'm trying to be cautious).

There are several ways of finding a derivative in this situation. The simplest way, or at least the one you learn first, is to introduce the idea of partial derivatives. First you ignore the fact that you can travel in the y direction and just try traveling in the x direction. The slope in that direction gives you the partial derivative of z with respect to x:



We use a curly 'd' so we know there are other things that could also cause the z value to vary, not just x.

You can also find the slope in the y direction, pretending x doesn't change and just seeing how fast z changes as you increase y. The two partial derivatives together can be used to give you general information about the slope of the function at that point in any given direction.

In the specific example of the car, we could see how the amount of petrol being used changes as the speed is varied, for a given weight carried, by taking the partial derivative of the amount of petrol being used with respect to changes in speed. Or if we wanted to know how fast the amount of petrol being used would change as we changed the weight carried, for a given speed, we'd take the partial derivative of petrol with respect to weight.

The Chain Rule

One last thing. The chain rule. In the ordinary case where you just have a function y that depends on a single variable x, it goes like this. Suppose x is also a function of some variable t. So for any given value of t, we can put that into a function to work out the value of x, and then we can put that x value into a different function to give us y.

t --> x --> y

The chain rule exists to deal with the following question. Suppose we know how fast x changes as t changes, and suppose we know how fast y changes as x changes. How fast does y change as t changes? The chain rule can tell us.

In the case where you have a function depending on two variables, it gets more complicated. You can have z depending on x and y, which both depend on t -- so for any given t value there will be x and y values which will then give you a specific value of z for that t value -- so there's a 'chain rule' to work out the derivative of z with respect to t based on the partial derivative of z with respect to x and y, and the derivatives of x and y with respect to t. Or it's not uncommon to have x and y both depending on the same pair of variables u and v. In that case, you might want to work out the partial derivative of z with respect to u or to v. The chain rule is easily adapted to let us use the partial derivatives of x and y with respect to u or v to tell us.

I haven't told you how to actually calculate any of this. (In keeping with that, I haven't told you in this post what the chain rule actually is!) Largely, I haven't told you that part of things because becoming proficient at the calculational side takes practice. This means you won't be able to understand the calculations in my post above. You might get an inkling of the concepts, though.

Subjectivity

If there's a God, or any kind of justice under the sky,
If there's a point, if there's a reason to live or die,
If there's an answer to the questions we feel bound to ask,
Show yourself! Destroy our fears! Release your mask!

Oh, yes, we'll keep on trying . . .

-- Queen, Innuendo (Despite the title, that song contains little or no sexual content, and, indeed, no content more offensive than the above.)

I understand why theists use the "if that was true life wouldn't be worth living" argument. Sure, it doesn't actually have any effect on the facts of the case. Maybe life just isn't worth living. But I know full well that if I thought believing something would make me want to kill myself, I would hesitate on the objectivity a little. More, I've got a moral sense as strong and passionate as any and if I thought believing something would make me do something seriously morally wrong, well, I might allow myself a little self-deception. Of course, you'd be a fool to expect something you believe because you can't live without believing it to be a reliable indicator of the sorts of conclusions you'd come to based on actual evidence. No doubt that's where the 'non-overlapping magisteria' rule comes from. It makes sense, when you think about it. Go ahead and believe in God if you need to, says that rule, but don't come whining when the fossil evidence contradicts the petty details of the structure you've taken on in an attempt to find purpose. There's no reason to expect agreement.

In atheist attempts to avoid making statements about the world which are not supported by evidence, I suspect some believers occasionally hear us as saying "Question everything, and discard anything that doesn't correspond to an objective phenomenon of the world outside our minds". That's not what we're saying. Indeed, that would be nihilism. Love is not an objective phenomenon of the world outside our minds, and nor is happiness.

At other times, while they understand atheism might not ask us to stop caring per se, believers might be afraid that they would stop caring if they didn't have the justification.

Does it scare you to consider the possibility that the care you feel is only in your mind, not justified by any certain logic of the world outside you? Then take yourself to the brink. Go there because it is a rite of passage, go there by reciting truths. The universe doesn't care if you live or die. You could kill yourself now, and the effect on the world in two hundred years might be large, but only by chaos theory, not by any effect that a historian could trace. You don't have to care. It would be just as logical not to. Say it and feel it and go to the brink and watch the nothingness bubble. It burns a little; maybe a lot; maybe it takes genuine care for the truth to force you to risk it. And it bubbles. You can't help it bubbling. Little bits of reality or imagination will insist on popping up, no matter how hard you try. So no-one will care in two hundred years. But people care now, don't they? It's a reflex, I think, you find yourself resurfacing for the sake of survival. Squash it. I dare you. But you're only allowed to use true statements. How about "But they don't have to care"? How about "But there's no reason to care if they care"? I bet you still do care. Can you make yourself stop? I can't. On this count, and on several others, the bubble just won't pop. The truth is not enough to sever you from your ability to care. It never could be.

You can go to the brink, but you take your humanity with you.

Now, here's the big question. Do you have to stop? Does caring about anything mean that you've abandoned the principles of truth that atheists claim to value? Are you believing anything false by caring? Well, if you ask me, the process of caring is not logically connected to the process of believing at all. Sure, there's a connection between what you believe and what you care about. If you believe your sister cares about you, for example, you might be more likely to care about her. But that's not a logical connection. It might perhaps be "little-'o' objective"[1]; that's about the best you can do. There's really no logical connection either way. You don't logically have to care. You don't logically have to not care.

The rule isn't "Discard anything that can't be shown to correspond to an objective phenomenon of the world outside our minds". In my opinion, the rule is actually "Don't call anything an objective phenomenon without some evidence that it actually is one". That doesn't mean you're not allowed to care about the subjective or, indeed, to care subjectively.

You can't leave your subjectivity behind. And you don't have to. Aren't you glad?

Continued, because I've thought again and I don't like this post as it is:

I'm aware that there's another issue here. Can you be satisfied with the truths you have? Is it enough to know that you're here now, even if you don't have eternity? Is it enough for you that there are people you love and who love you -- is it enough to know that you may have to work to keep the real things in your life because you won't necessarily have God -- is it enough for you to care, well, just because you do?

I think, for most people, it is enough. I think there are far more people who think they can't do this than people who really can't. They haven't ever had to build a life based on things that a skeptical atheist can accept, and they just don't know how much there is to work with. But I've seen the nothingness and felt it burn and if running from it makes you run from atheism, well, I'm not going to shout at you and call you stupid. I'd rather just offer my hand.

[1] I borrow this terminology from Steve Novella here; thanks are due to Ordinary Girl in the comments of this post here on Elliptica for pointing me towards it. By "little-'o' objective" I mean based not on some objective fact of the world outside our minds but rather on some aspect that is near-universal within our minds.